Consecutive Angles: Definition, Types, Solved Math Tasks
reviewed by Jo-ann Caballes
Updated on November 20, 2024
Geometry is one of the trickiest school subjects, so no wonder that so many students ask questions such as “Which angles are consecutive interior angles?” and why those angles are so important.
That’s why we, at Brighterly, created this in-depth guide for you to understand consecutive geometry and why it’s simpler than it seems to be.
What Are Consecutive Angles?
Consecutive angles are a pair of angles formed on each side of the transversal when it interacts with two parallel lines. The angles in the same region (either interior or exterior) on each of the parallel lines form consecutive angle pairs. Consecutive angles are supplementary, meaning that the sum of both angles is equal to 180 degrees
It may sound scary, but once you look at the illustration, you’ll understand the consecutive angles meaning.
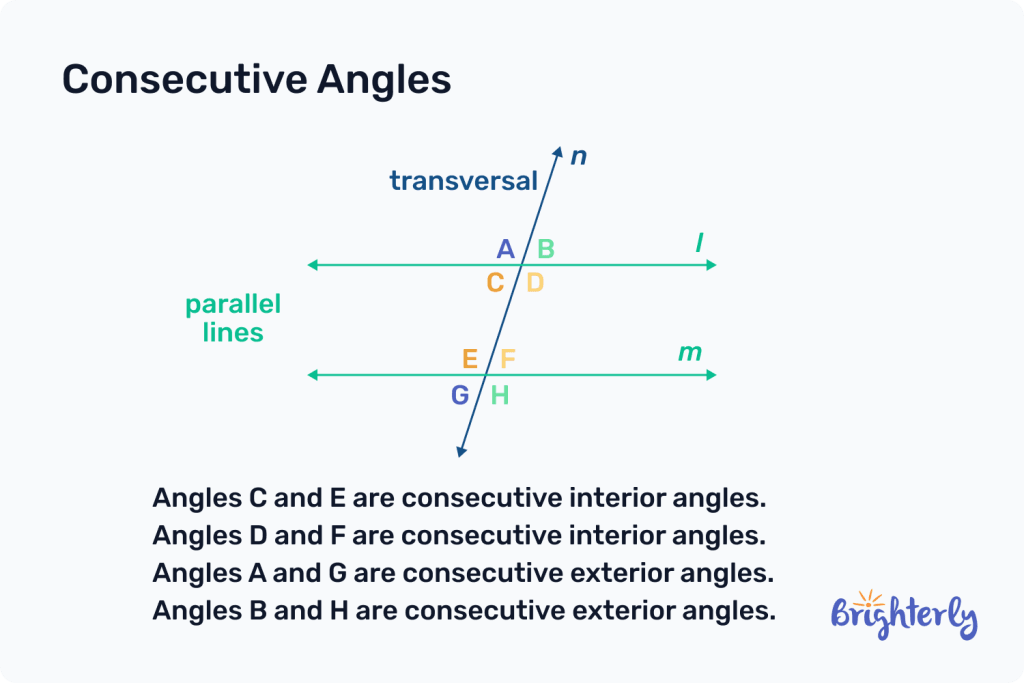
Consecutive Angles: Definition
Speaking more scientifically, consecutive angles are a pair of angles formed when a transversal intersects two parallel lines. They are located on the same side of the transversal and can be either interior or exterior angles. There are two types of a consecutive angle: interior and exterior.
Consecutive Angles: Types
There are two primary types of consecutive angles: interior and exterior. Interior ones are a pair of angles that lie between the two parallel lines and on the same side of the transversal. Exterior angles are a pair of angles that lie outside the two parallel lines and on the same side of the transversal.
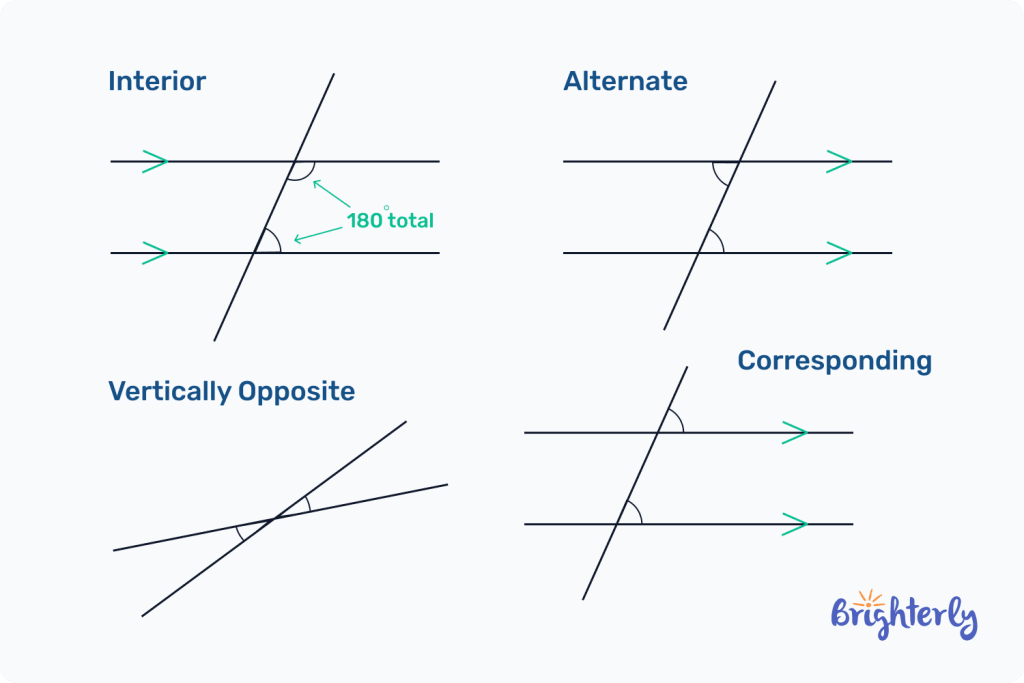
The key to identifying consecutive geometry is that those angles must be on the same side of the transversal. Their position within or outside the parallel lines determines whether they are interior or exterior.
Consecutive interior angles: Definition
Consecutive interior angles are a pair of angles formed when a transversal intersects two parallel lines. They are located on the same side of the transversal and are inside the two parallel lines. They are also known as co-interior angles or same-side interior angles.
The key property of consecutive interior angles is that they are supplementary, meaning their sum is always 180 degrees. This is known as the Consecutive Angles Theorem.
This theorem is a fundamental concept in geometry and is often used to prove other theorems and solve problems involving parallel lines and angles.
Example of consecutive interior angles
If you are still trying to understand this geometry, let’s review examples of consecutive interior angles. In the diagram below, we have two parallel lines, s and t, intersected by a transversal. This configuration creates several pairs of consecutive angles.
There are 2 pairs of interior angles: C and F, D and E. They lie between the parallel lines and on the same side of the transversal. Meanwhile, A and H, B and G are consecutive exterior angles.
The sum of the measures of consecutive angles is always 180°. In this case, ∠c + ∠f = 180°. At the same time, ∠d + ∠e = 180°. The same applies to exterior angles.
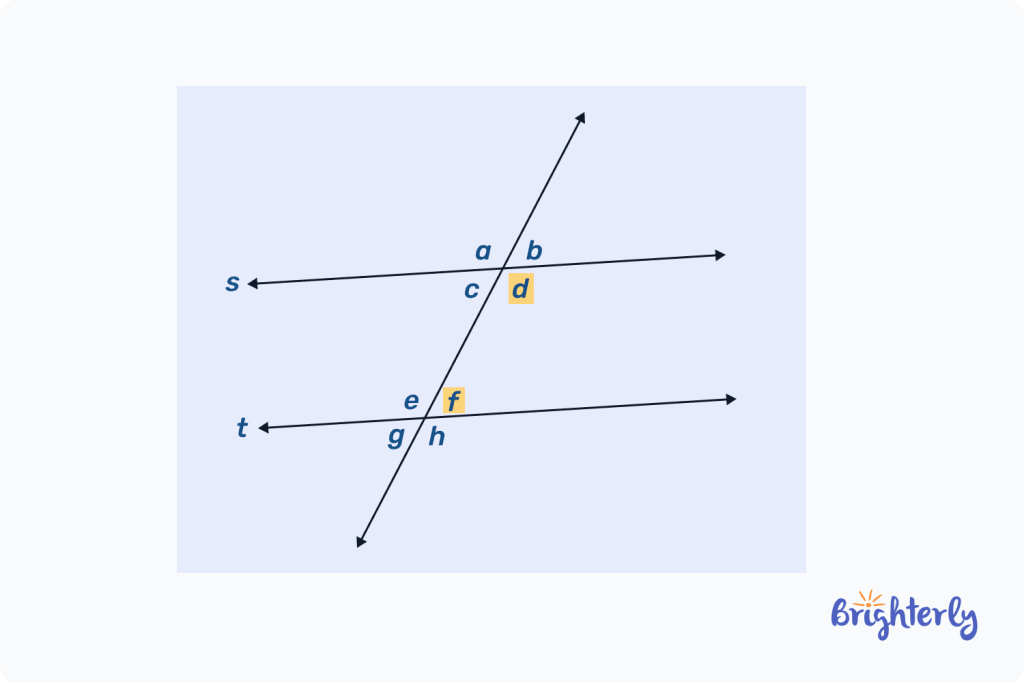
Consecutive Interior Angles Theorem
The Consecutive Interior Angles Theorem states that if a transversal intersects two parallel lines, then each pair of consecutive interior angles is supplementary. In simpler terms, this means that when a line crosses two parallel lines, the two inner angles on the same side of the transversal add up to 180 degrees.
If angle 1 is 70°, then angle 2 must be 110° because 70 + 110 = 180.
Consecutive Angles in a Parallelogram
The consecutive angles parallelogram theory is easy since any two consecutive angles are supplementary. The sum of the measures of any two adjacent angles in a parallelogram is always 180 degrees.
When you draw a diagonal in a parallelogram, you create two pairs of alternate interior angles. These pairs of angles are congruent. Since alternate interior angles are supplementary, it follows that consecutive angles of a parallelogram are also supplementary.
Solved Math Tasks: Examples
Solved math problem 1
If one consecutive exterior angle measures 115 degrees, what is the measure of the other consecutive exterior angle?
Answer
To calculate consecutive exterior angles, you should recall that they are supplementary, meaning they add up to 180 degrees. So, if one angle is 115 degrees, the other angle is:
180 degrees – 115 degrees = 65 degrees
|
Therefore, the measure of the other consecutive exterior angle is 65 degrees. |
Solved math problem 2
Problem: In the figure below, line l || m. Prove that line p || q.
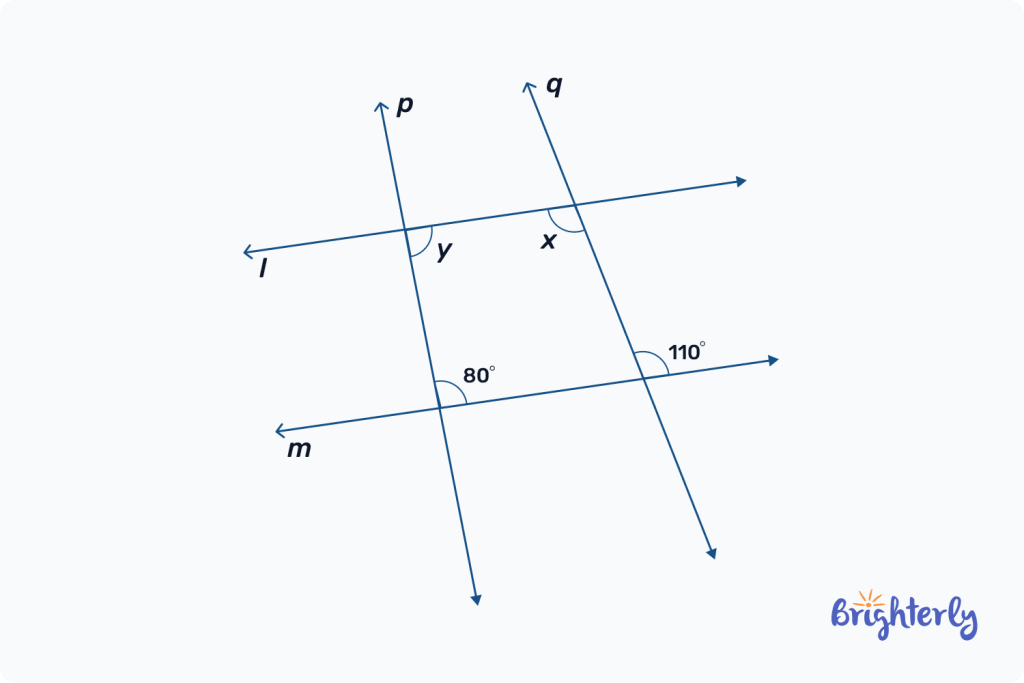
Answer
The first thing that you need to recall is to ask yourself whether consecutive exterior angles are congruent or supplementary. Since they are supplementary, there will be 2 consecutive couples.
You have 4 angles here, and since l || m, we know that Angle 1 and Angle 2 are supplementary due to the consecutive interior angles theorem.
Angle 1 and Angle 3 are vertical angles, so they are congruent. Angle 2 and Angle 4 are also vertical angles, so they are congruent too. So, if Angle 1 and Angle 2 are supplementary, Angle 1 is congruent to Angle 3, and Angle 2 is congruent to Angle 4, then Angle 3 and Angle 4 must also be supplementary.
Now you can apply the converse of the Consecutive Interior Angles Theorem. If two lines are intersected by a transversal so that a pair of consecutive interior angles are supplementary, then the lines are parallel.
| Therefore, line p || q. |
Consecutive Angles: Practice Math Problems
Consecutive Angles: Worksheets
Now that you know the consecutive angles meaning and how to use them, it’s time to practice geometry with the worksheets. Use Brighterly worksheets to boost your geometry skills. Consecutive angles math is crucial for any geometry figure, so you can test the math knowledge of your child with these geometry worksheets:
Missing Angles In Triangles Worksheets
Properties Of Rectangles Worksheets
Math homework help for grade 1-8
Get the homework support your child needs!
Choose kid's grade
Math Program
Boost Your Child's Math Abilities! Ideal for 1st-8th Graders, Perfectly Synced with School Curriculum!
