Numerical Expression – Definition with Examples
reviewed by Jo-ann Caballes
Updated on December 19, 2024
Welcome to Brighterly, where we break down math concepts to make them simple to understand and exciting to learn.
Today, we’re going to cover a key concept – the numerical expression. These statements are foundational to many math concepts and equations.
Here, we’ll cover what is a numerical expression for 5th grade, how to write a numerical expression and numerical expressions examples. We’ll also share practice test questions and worksheets to take your learning further.
What is a numerical expression?
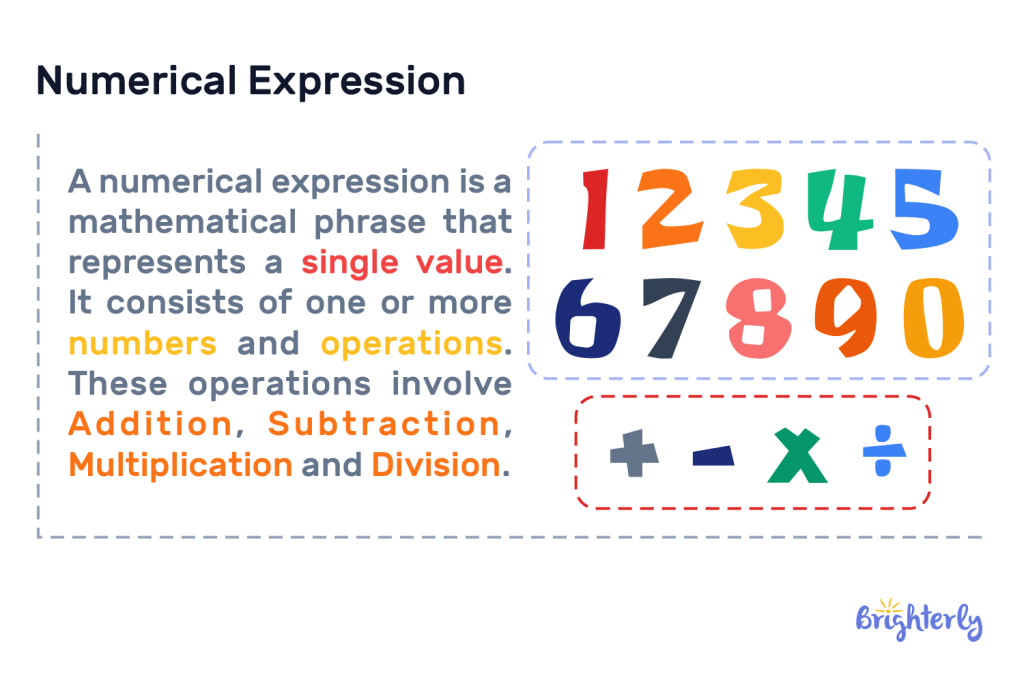
A numerical expression meaning is an expression that contains only numbers and operations. These items combine to form an expression. We define numerical expression in full below.
Numerical expression definition
The numerical expression definition in math is a statement that includes numericals and operations. Any numbers can be combined with any of the 4 operations – addition, subtraction, multiplication and division – and a numerical expression can have multiple numbers and operations.
Understanding the components
There are three key components of numerical expressions:
- Numbers – they can be integers, decimal numbers or fractions
- Operations – addition, subtraction, multiplication and division
- Parentheses – used for grouping parts of your expression
How to write a numerical expression?
To write a numerical expression, you combine numbers, operations and parentheses (if required). As an example of numerical expression, if you were adding 2 and 3, your expression would be 2 + 3. There is no limit to how many numbers or operations you can use in a numerical expression. You can also write the numerical expression in words – for example, two plus three.
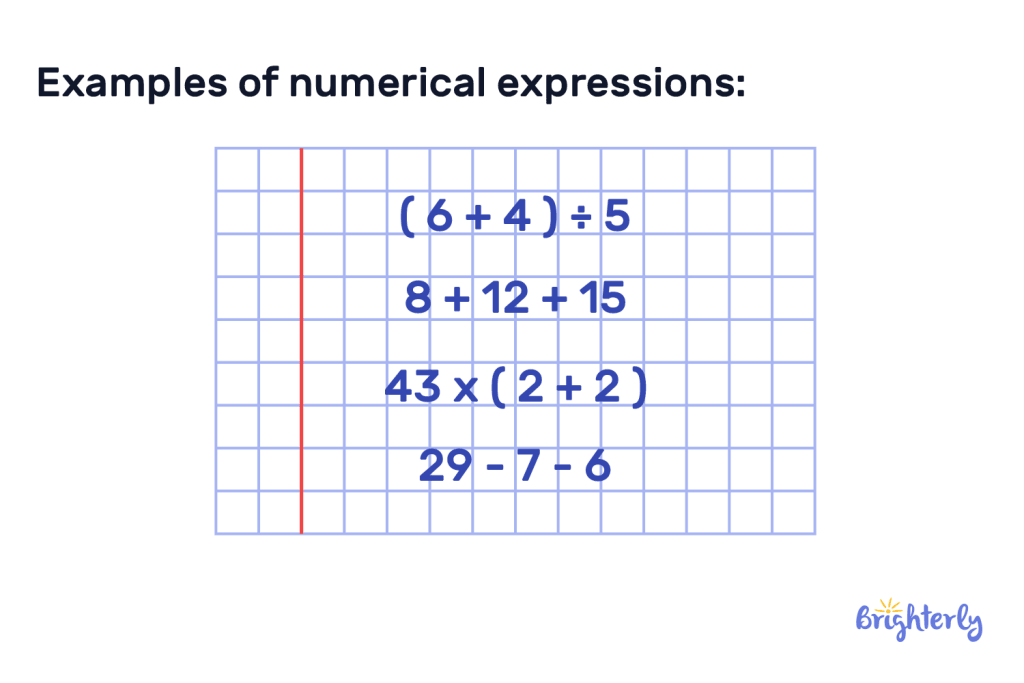
Examples of numerical expressions
Here are some numerical expression examples:
- (6 + 4) ÷ 5
- 8 + 12 + 15
- 43 x (2 + 2)
- 29 – 7 – 6
Why are numerical expressions important?
Numerical expressions are important because they form the basis of many different math concepts. We use expressions in our everyday lives, such as working out how much change we’ll get from our shopping or counting sets of items. Numerical expression in words can also help us understand math problems.
Importance of parentheses in numerical expressions
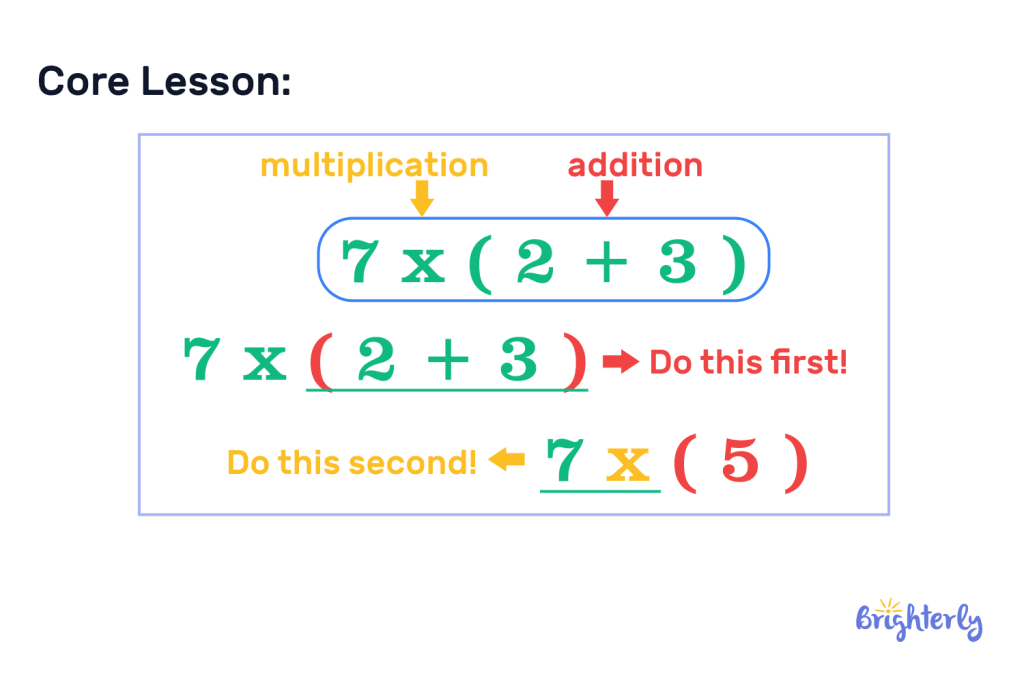
Parentheses aren’t always used in numerical expressions, but when they are, they perform an important function. They tell us which part of the expression we need to solve first. As a numerical expression example, if we have the expression 13 + (4 – 2), we know we need to solve 4 – 2 before anything else. 4 – 2 = 2, so our expression can therefore be simplified to 13 – 2 = 11.
If this expression didn’t have parentheses, the outcome would be different: 13 + 4 – 2 = 15.
Real-life applications of numerical expressions
Numerical expressions have a range of real-life applications, including:
- Adding up items in your shopping basket
- Calculating sales tax on items
- Working with a budget
- Measuring ingredients when cooking or baking
Solved examples of numerical expression
Now that you understand what a numerical expression is and how to write it, it’s time to try out some practice puzzles. Work out the answers to our examples, then check the solutions to see how many you got right.
Solved math example 1
Solve the following expression:
35 + (10 – 3)
Answer:
| 42. |
First, we solve 10 – 3 = 7.
Then, we add this to 35. 35 + 7 = 42.
Solved math task 2
Solve the following expression:
95 + 12 – 23
Answer:
| 84. |
Because there are no parentheses in this expression, we solve it chronologically. 95 + 12 = 107. 107 – 23 = 84.
Solved math task 3
Solve the following expression:
5 x (10 ÷ 5)
Answer:
| 10. |
Because this expression has parentheses, we solve that part first. 10 ÷ 5 = 2. Therefore, our expression becomes 5 x 2 = 10.
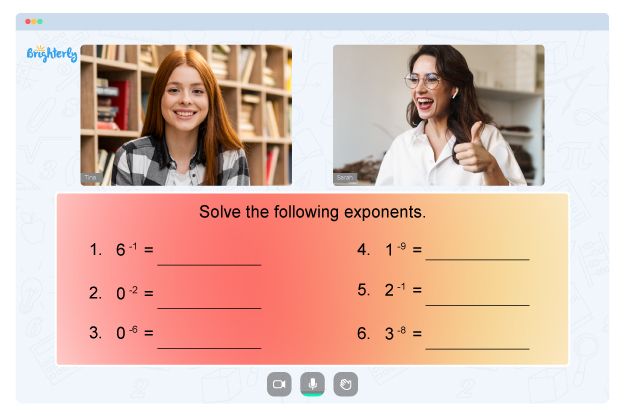
Numerical expression problems: practice
Frequently asked questions
What is the difference between a numerical expression and an algebraic expression?
The difference between a numerical expression and an algebraic expression is that a numerical expression only contains numbers, operations and sometimes parentheses. Meanwhile, an algebraic expression can also contain variables – letters that represent unknown numbers.
Can numerical expressions have more than one solution?
No, numerical expressions cannot have more than one solution. Because all numbers are already represented as themselves and not variables, there can only be one answer to numerical expressions.
How can I simplify a numerical expression?
You can simplify a numerical expression by performing the operation within the parentheses first if there is one. Once you’ve performed that operation, you have a shorter and more simplified numerical expression.
Are there any online resources or tools to practice numerical expressions?
Brighterly offers a range of online resources and tools to practice numerical expressions. You can use our practice test questions above or download our worksheets below to practice your learning on numerical expressions.
Can numerical expressions be used to solve real-life problems?
Yes, numerical expressions can be used to solve real-life problems. They can help you add up shopping lists, calculate sales tax, work with measurements in cooking and much more.
Numerical expression: worksheets
Want to improve your knowledge of numerical expressions even further? Try out our fun math worksheets to put your skills to the test and become a master of numerical expressions!