Perimeter of Rectangle
Updated on January 6, 2024
Welcome to Brighterly – your go-to resource for making math fun and engaging for children. Today, we will be exploring the concept of the perimeter of a rectangle. This important geometric concept allows us to analyze the properties and measurements of rectangles in a more comprehensive manner. In this blog post, we will delve into the formula, derivation, and relationship between the perimeter of a rectangle and other geometric shapes. Additionally, we will provide some solved examples and practice problems that will help young learners better understand and apply the concept. So without further ado, let’s jump right in!
What is the Perimeter of a Rectangle?
The perimeter of a rectangle is a fundamental concept in mathematics that refers to the total length of all its sides. To put it simply, the perimeter is the distance around the outer edge of the rectangle, forming a closed loop. This concept plays a crucial role in various areas of mathematics, including geometry and algebra, as it allows us to understand the properties of shapes and their relationships with one another. By learning about the perimeter of a rectangle, young minds can develop their problem-solving skills and gain a deeper appreciation for the beauty and complexity of mathematics.
At Brighterly, we strive to make learning enjoyable and accessible for children. By exploring the concept of the perimeter of a rectangle, we aim to spark curiosity and encourage young learners to think critically about the world around them. In the following sections, we will provide a comprehensive overview of the formula, derivation, and relationship between the perimeter of a rectangle and other geometric shapes. Furthermore, we will offer solved examples and practice problems that will help children apply their newfound knowledge and develop a strong foundation in mathematics.
Perimeter of a Rectangle Formula
The formula for the perimeter of a rectangle is given by:
P = 2(l + w)
where:
- P is the perimeter
- l is the length of the rectangle
- w is the width of the rectangle
This formula helps us calculate the perimeter by adding the length and the width of the rectangle and then multiplying the sum by 2.
Derivation of Perimeter of Rectangle
Let’s derive the formula for the perimeter of a rectangle. A rectangle has four sides, with the opposite sides being equal in length. Therefore, we have two pairs of equal sides: one pair with length ‘l’ and the other pair with width ‘w’.
To find the perimeter, we add the lengths of all four sides:
P = l + w + l + w
By rearranging the terms, we get:
P = 2l + 2w
Factoring out 2, we get the final formula:
P = 2(l + w)
Perimeter of Rectangle Formula vs Perimeter of Square Formula
A square is a special type of rectangle where all four sides are equal in length. In other words, a square is a rectangle with length (l) equal to its width (w). The formula for the perimeter of a square can be derived from the rectangle’s perimeter formula by substituting l for w:
Perimeter of Square = 4l
Comparing the two formulas, we can see that the perimeter of a square is four times its side length, while the perimeter of a rectangle is twice the sum of its length and width.
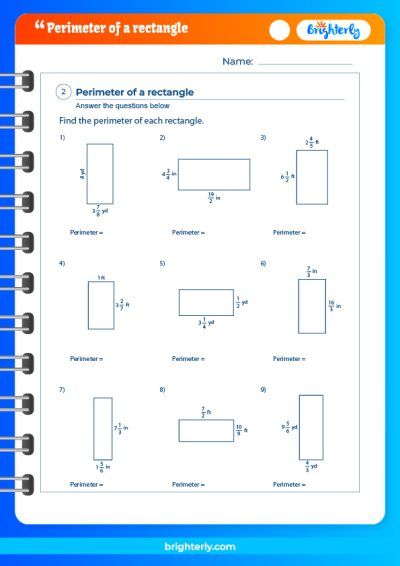
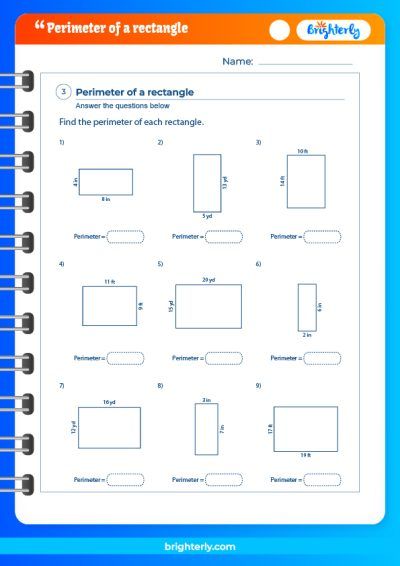
In order to better master this topic, we recommend that you pay attention to the math worksheets from Brighterly. They will allow you to learn and consolidate your knowledge of the topic “Perimeter of a rectangle”.
Relationship Between Area and Perimeter of a Rectangle
The area of a rectangle is the amount of space enclosed by its sides, while the perimeter is the total length of its sides. The formulas for the area and perimeter of a rectangle are related but distinct:
- Area (A) = length (l) × width (w)
- Perimeter (P) = 2(l + w)
While there is no direct formula to calculate the area from the perimeter or vice versa, knowing one of these measurements can help in solving problems related to the other, given additional information.
How to Find the Perimeter of a Rectangle?
To find the perimeter of a rectangle, follow these simple steps:
- Measure the length (l) and width (w) of the rectangle.
- Add the length and the width: l + w.
- Multiply the sum by 2: 2(l + w).
- The result is the perimeter of the rectangle.
Usage of Perimeter of a Rectangle
The concept of the perimeter of a rectangle is widely used in everyday life and various fields, including architecture, landscaping, and design. For example, you may need to calculate the perimeter to determine the amount of fencing required for a rectangular garden or to measure the dimensions of a room for installing baseboards.
Solved Examples On Perimeter of Rectangle
Example 1: A rectangular field has a length of 60 meters and a width of 40 meters. Find the perimeter of the field.
Solution: Using the formula for the perimeter of a rectangle, P = 2(l + w):
P = 2(60 + 40)
P = 2(100)
P = 200 meters
The perimeter of the field is 200 meters.
Example 2: A rectangular swimming pool has a length of 25 meters and a width of 15 meters. Calculate the amount of fencing needed to surround the pool.
Solution: To find the amount of fencing needed, we must first calculate the perimeter of the swimming pool using the formula P = 2(l + w):
P = 2(25 + 15)
P = 2(40)
P = 80 meters
The amount of fencing needed is 80 meters.
Practice Problems On Perimeter of Rectangle
- A rectangular garden has a length of 20 meters and a width of 10 meters. What is the perimeter of the garden?
- A picture frame has a length of 30 centimeters and a width of 20 centimeters. Calculate the perimeter of the frame.
- A rectangular rug has a perimeter of 36 meters, and its length is 12 meters. What is the width of the rug?
Conclusion
As we conclude our exploration of the perimeter of a rectangle, we at Brighterly want to emphasize the importance of understanding this fundamental geometric concept. By delving into the intricacies of the rectangle’s properties and applications, young learners can develop a strong foundation in mathematics that will serve them well in various aspects of life.
Our goal at Brighterly is to make learning enjoyable, engaging, and accessible for children. Through this comprehensive blog post, we have aimed to provide valuable insights and unique perspectives on the concept of the perimeter of a rectangle. By discussing the formula, derivation, and relationships with other geometric shapes, as well as offering solved examples and practice problems, we hope to inspire curiosity and foster a love for mathematics in young minds.
In conclusion, the perimeter of a rectangle is an essential concept that plays a crucial role in understanding the properties and applications of geometric shapes. By mastering this concept, young learners will be better equipped to tackle more complex mathematical problems and develop a deep appreciation for the beauty and complexity of mathematics. We hope that this blog post has provided you with valuable insights and that you have enjoyed learning with Brighterly. Keep exploring, and remember that the world of mathematics is full of exciting discoveries waiting to be made!
Frequently Asked Questions On Perimeter of Rectangle
What is the difference between the perimeter and the area of a rectangle?
The perimeter of a rectangle refers to the total length of its sides, while the area is the amount of space enclosed by its sides. The formulas for the area and perimeter of a rectangle are related but distinct.
Can I find the area of a rectangle if I know its perimeter?
Knowing the perimeter alone is not enough to determine the area of a rectangle. You will need additional information, such as the length or width, to calculate the area.
Is the perimeter of a rectangle always greater than its area?
No, the perimeter of a rectangle is not always greater than its area. The relationship between the area and perimeter depends on the dimensions of the rectangle.