• Math tutors
• /
• Knowledge Base
• /
• What is radius of a circle? Definition, Formula, and Examples

What is radius of a circle? Definition, Formula, and Examples

Jessica Kaminski

reviewed by Jo-ann Caballes

Updated on November 15, 2024

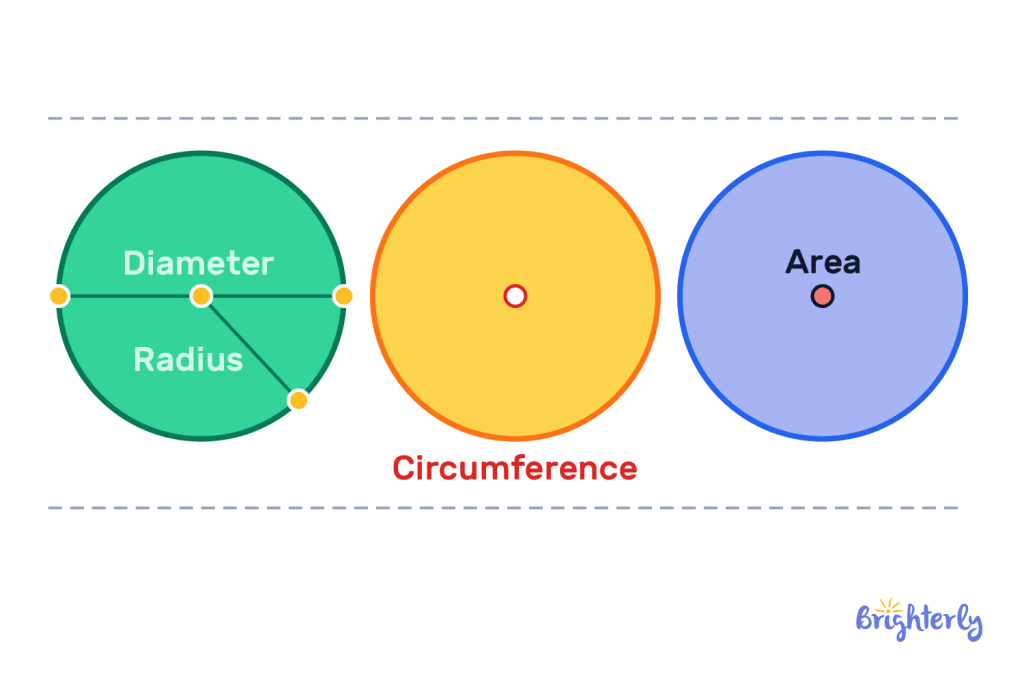
The circle is made up of the circumference, diameter, center, and radius. 

Our interest today is in the radius. We look at the definition of a radius and the radius of a circle definition, explain radius terms, and give formulas to help you find the radius, alongside examples and practice questions. We also recommend worksheets by our expert tutors to help students understand this element of a circle. 

What is a radius?

A radius is the distance within a circle from its center to any point in its outer boundary which is called its circumference. 

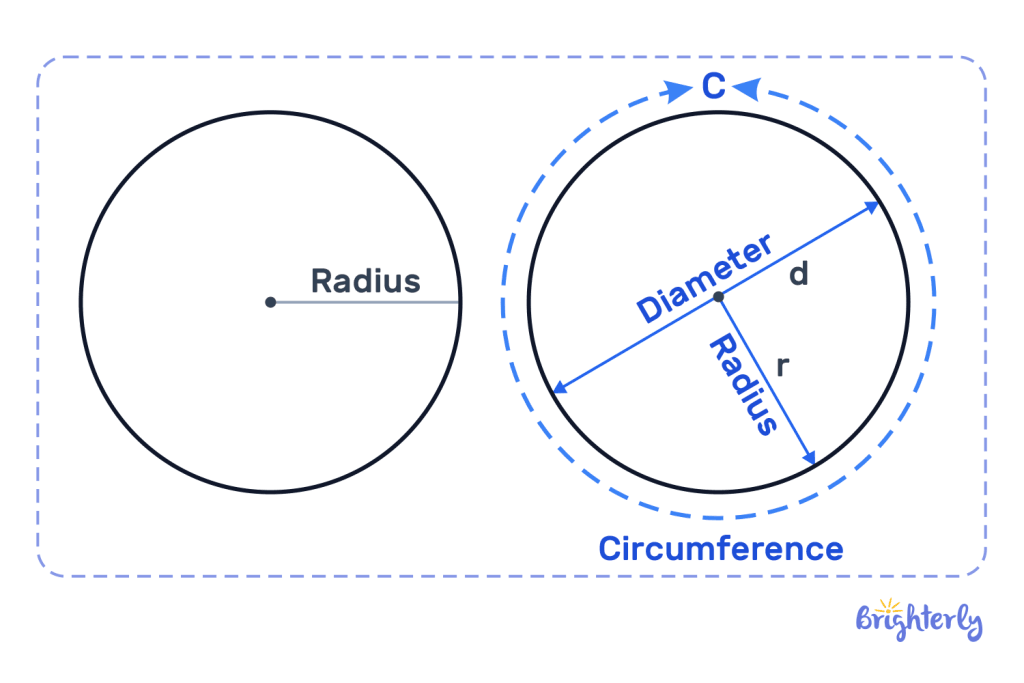
Radius definition in geometry

We can define radius in math and geometry as a line segment that starts in the middle of a circular figure and ends at any point in the shape.

Note that the radius is not unique to circles or 2-dimensional shapes alone. Radius can also be found in some 3-dimensional shapes and shapes with circular bases such as the sphere, cylinder, and cone.

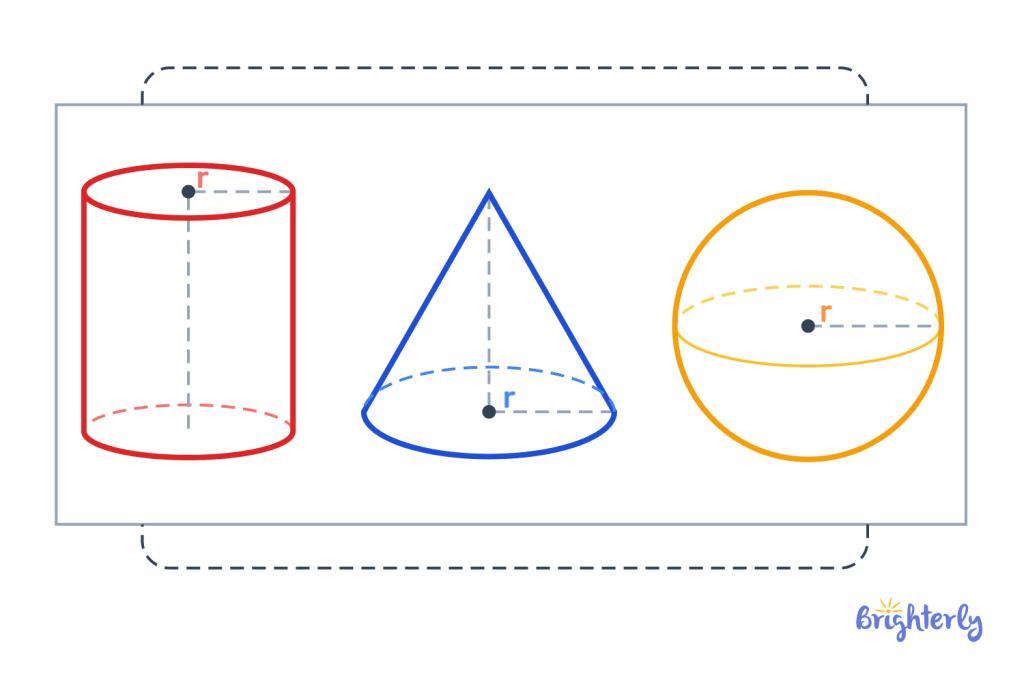
Radius examples

You can find a radius example in real-life objects such as:

• A toilet paper roll
• Balloons
• An ice cream cone
• Coins
• Bicycle wheels, and so on.

You can find the radius of any and all of these objects by tracing a line from the middle to any point in the circle or the circular base.

What is the radius of a circle?

As a circle is a 2-dimensional shape, we may define the radius of a circle as a line segment that starts with an endpoint from the center and another endpoint at the edge of the circle.

Math tutors

Join Brighterly for personalized lessons for your child

Meet the best tutors for interactive math learning.

Book free lesson

Radius of a circle example

We can find the radius in real-life circular examples such as:

• A pizza 
• Tire wheels 
• Round table tops
• Round wall clocks

The radius of a circle: Formulas 

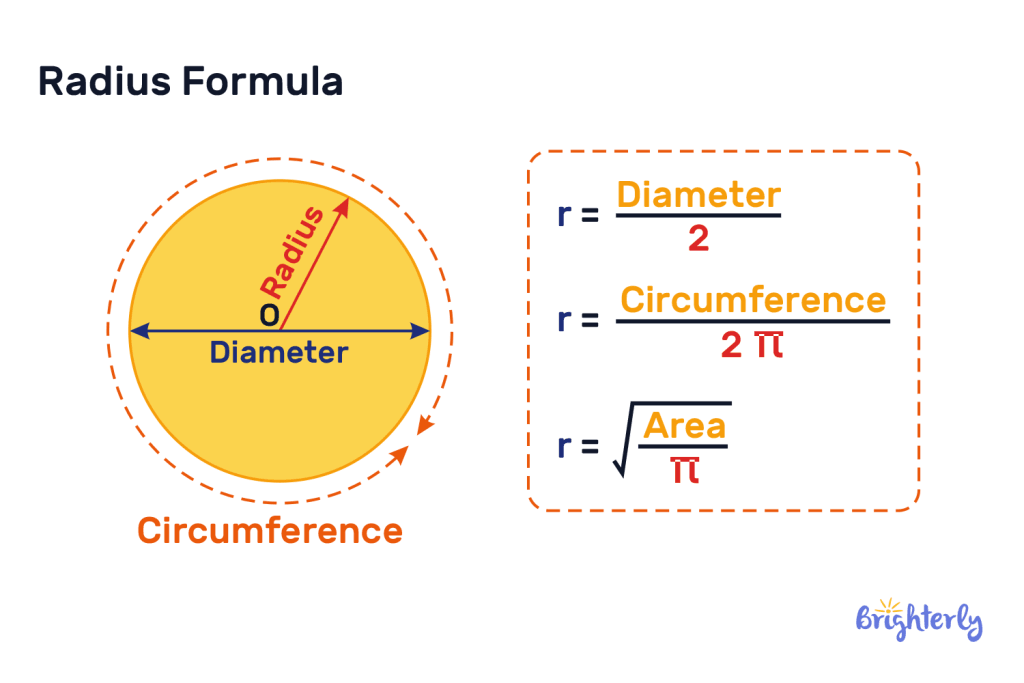
We can determine the radius of a circle through more than one formula — we can use the area, diameter, and circumference of the circle.

Formula for radius using the area 

The area of a shape is the amount of space it occupies or the amount of space within the shape. This also applies to the circle. 

We find the area of a circle by multiplying pi (π) by the square of the radius:

Area = π × r 2

And the radius of a circle is the square root of the area divided by pi: 

Radius = √Area/ π 

Formula for radius using the diameter

The diameter is the longest length in a circle. It is the distance from one end of the circle to another and it passes through the center. 

The diameter is double the radius:

Diameter = 2 × radius 

This also means we may find the radius of a circle by dividing the diameter by 2:

Radius = Diameter ÷ 2.

Formula for radius using the circumference

The circumference is the total boundary that surrounds the circle. We find the circumference by multiplying pi by the diameter of the circle:

Circumference = π × d

With the diameter, we can find the radius by dividing the circumference by 2 multiplied by pi:

Radius = C/2π 

The radius of a circle: Equation

It is possible to find the equation of a circle when we know the radius. If the coordinates for the  center of the circle are h and k, we can express the equation as:

(x − h)2 + (y − k)2 =r2

Solved Math Tasks: Examples

Solved math problem 1

A circle has a circumference of 24 units. What is the radius of the circle?

Answer 

We know that to find the radius using the circumference, we divide it by the result of 2 multiplied by pi which is:

2 × 3.14 = 6.28

Radius = 24 ÷ 6.28

Solved math problem 2

Jake’s soccer ball has a surface area of 1,232 centimeters, what is the radius?

Answer 

With the formula we discussed earlier, we can find the radius by:

Radius = √1232/ π 

= √392.35 = 19.8 cm

The radius of a circle: Practice Math Problems

The radius of a circle: worksheets

Learn more about the radius and other parts of a circle with Brighterly’s free worksheets;

• Parts of a circle worksheets 
• Circle worksheets 
• Area of a circle worksheets 

Jessica Kaminski

70 articles

Jessica is a a seasoned math tutor with over a decade of experience in the field. With a BSc and Master’s degree in Mathematics, she enjoys nurturing math geniuses, regardless of their age, grade, and skills. Apart from tutoring, Jessica blogs at Brighterly. She also has experience in child psychology, homeschooling and curriculum consultation for schools and EdTech websites.